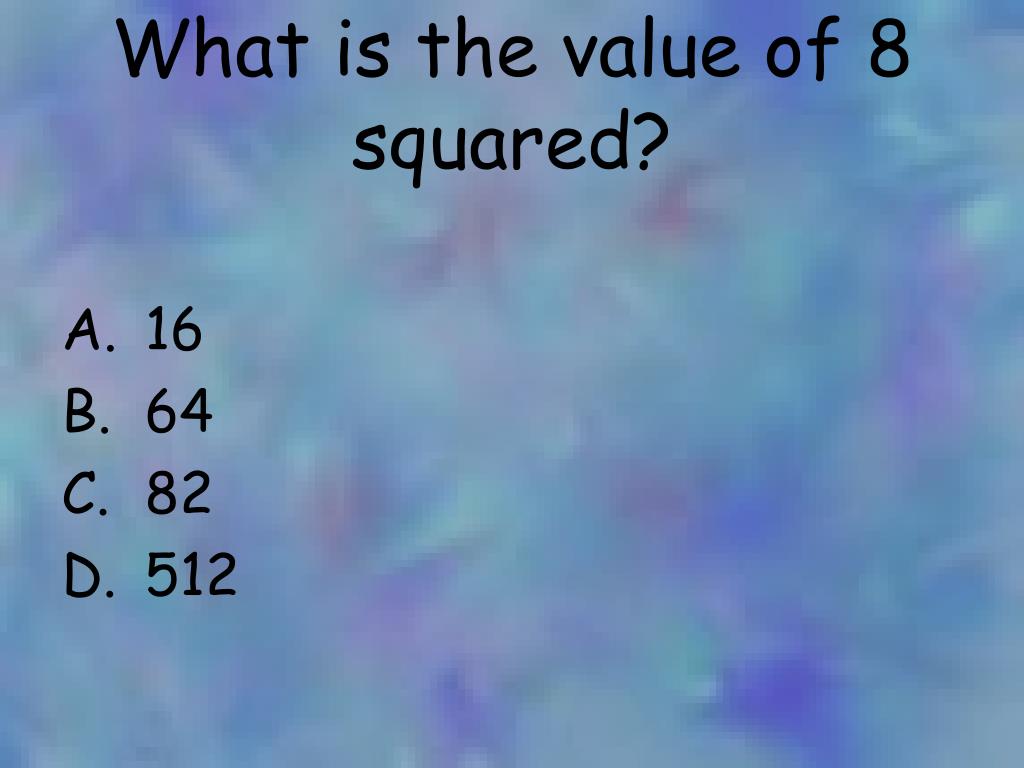Find Any Number's Square: Easy Calculator + Examples!
Ever wondered how a simple multiplication can unlock a world of mathematical possibilities? Understanding the concept of "squared" values is fundamental to grasping more complex mathematical ideas, and it's surprisingly simple to grasp.
Let's delve into the essence of squaring a number. At its core, squaring a number means multiplying it by itself. For instance, the phrase "8 squared" or "8 to the power of 2" translates to 8 8. This operation results in a single value: 64. This is a fundamental mathematical process, and this operation is the bedrock for more advanced concepts.
This concept extends far beyond simple arithmetic. The area of a square, for instance, is calculated by squaring the length of one of its sides. If a square has sides each measuring 8 units, its area is 8 8 = 64 square units. This connection between squaring and geometric concepts highlights the widespread relevance of this simple operation.
The beauty of squaring lies not only in its simplicity but also in its applicability. Whether you're working with positive or negative numbers, the principle remains the same: a number multiplied by itself. The result changes depending on the sign of the original number, but the process is always the same. Also, any online calculator can effortlessly compute the squared value of any given number, irrespective of whether it's a whole number or a decimal. For instance, you can input "8" into a calculator, and it will instantly return "64".
Furthermore, the concept of "squared" extends into the realm of exponents. When an exponent is 2, it indicates that a number is to be multiplied by itself. This is a concise way of expressing repeated multiplication. For example, 5 is the same as 5 5 = 25. This concept forms the basis for handling more complex equations and understanding exponential growth or decay in various scenarios, such as calculating compound interest or modelling population growth.
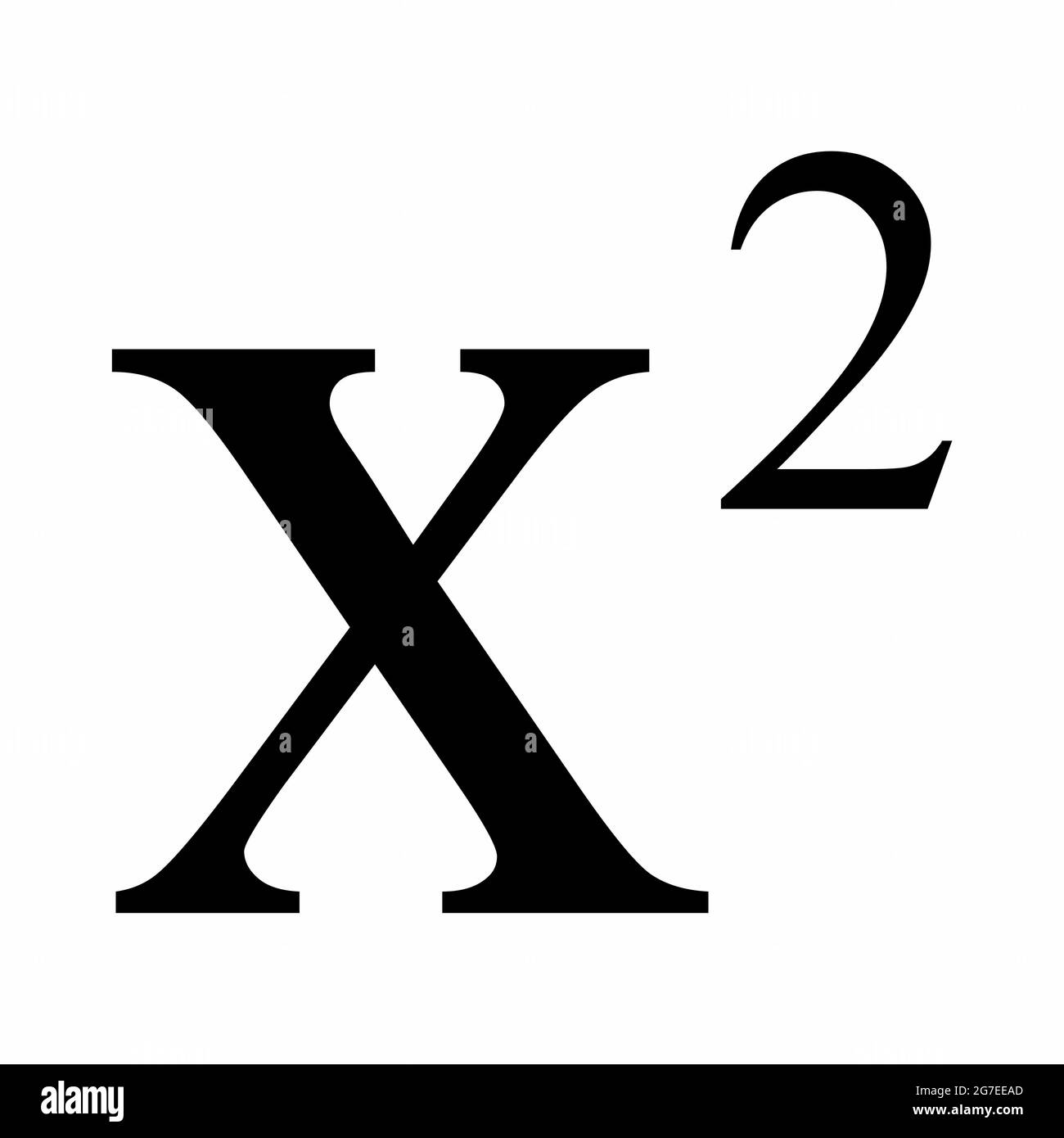
Beyond the basics, the squared symbol () is itself worthy of note. It's a shorthand for the exponent "2," and its use streamlines the expression of repeated multiplication. Similarly, you'll find superscript symbols (like , , , etc.) used in various mathematical notations. Being aware of these symbols, and how they relate to exponential calculations, provides a more comprehensive understanding of mathematics and the related symbolic language. You may find these characters in various forms: .
For the purposes of clarification, we should also note that squaring can involve negative numbers. The result of squaring a negative number is always positive because a negative times a negative equals a positive. For example, (-8) (-8) = 64. This simple rule of multiplication reveals a significant distinction.
Another interesting property of squared numbers is their relationship to odd numbers. The square of a number can also be expressed as the sum of consecutive odd numbers. For example, 8 is the same as the sum of the first 8 odd numbers: 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 = 64. This relationship between squared numbers and odd numbers adds a new layer of insight into their properties.
The usefulness of this mathematical operation extends into the practical world. In fields like engineering, physics, and finance, squaring is a commonplace operation. For instance, it is frequently used in calculating the area of various shapes, determining the energy of a moving object, or in financial modeling.
When faced with a problem that requires calculating a squared value, consider visualizing it in terms of a square. If the problem involves "8 squared," for example, imagine a square where all the sides are 8 units long. The area of this square is the same as 8 squared.
In summary, calculating the squared value of a number is the simple act of multiplying the number by itself. This foundational concept has wide applications across various mathematical branches and is an invaluable tool in many real-world applications. From calculating the area of a square to understanding exponents and their significance, "squaring" is more than a mathematical operation; it is the key to unlocking various complex concepts.
The factoring calculator aids in simplifying complex mathematical expressions.
It is a crucial process that is helpful in transforming intricate formulas into products of simpler factors.
The calculator works with polynomials and expressions, even those that contain various variables.
When squaring fractions, square both the numerator and denominator to get the answer.
For more complex calculations involving exponents, positive, negative, and zero exponents should all be studied.
In situations where you encounter difficulties loading the website's external sources, make sure that the domains .kastatic.org and .kasandbox.org are not blocked.
To simplify multiplications, learning about exponents and how to use them is important.
Exponents are crucial because they show how many times a number should be multiplied.